Tattoos

Don't fuck with the maths. I will hunt you down and kill you.

Well now, I may take this seriously if you can perform a full derivation of Euler's identity, form a suitably simple starting point, next time I see you.

otherwise, it's an empty threat since you don't really understand it anyway...

BTW, Emilé, I don't think I could do the above! It'd be fun to sit down and try it together though eh?

Euler is one of your favourite topics.

To make things interesting:

We should take a number of important areas of mathematics (Previously agreed upon) and placed them in a hat.
You can draw the topic.
Then we'll sit down and work through it.

NB. I'll let you include Euler in the options, potentially, you could even work out the probability of drawing it from the tifter.

^Don't make Kate stab you...

Euler is one of your favourite topics.

To make things interesting:

We should take a number of important areas of mathematics (Previously agreed upon) and placed them in a hat.
You can draw the topic.
Then we'll sit down and work through it.

NB. I'll let you include Euler in the options, potentially, you could even work out the probability of drawing it from the tifter.

This sounds like cool idea, definitely:

http://www.lfgss.com/post1509239-36.html

^Don't make Kate stab you...

Who is Kate?

Who is Kate?

Who is Euler?

Euler?

Euler?

Euler?

Eulerk like you could use an encyclopedia.

@ Mashton: Digger's keeper.

Some Object porn...

Citation?

Christ, don't ask me anything even vaguely mathsy!

If it's beyond ten fingers and ten toes then I'm fucked!

I'm words, not numbers.

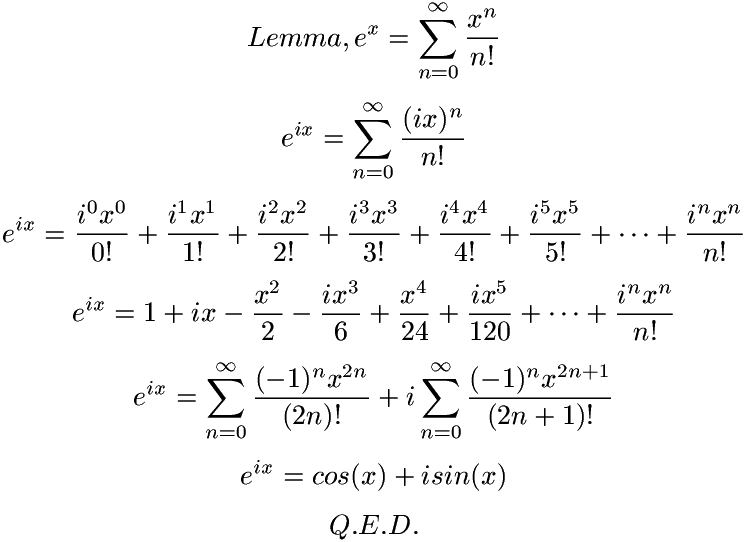
Incidentally, what does the above refer to?
Anyone? The 'formula'(?)that ends in Q.E.D, I mean.

Is it a recipe for cake?

I'm words, not numbers.

I'm pictures... where are the pictures people?!

I might get a tattoo just so I can post some pictures in this thread.

I'm pictures too.

Especially rudey pictures.

my legs
http://www.lfgss.com/attachment.php?attachmentid=27413&stc=1&d=1278426468

1 Attachment

Who's is the bike in the foreground? I'm pretty sure I saw someone on that the other day.

its Roy his bike, from Rotterdam, if you were in brussels the other day you could have seen it, otherwise it wasnot that bike

It's a derivation of Euler's Theroem, which allows you to express complex exponentials in terms of geometric functions. Which is really quite amazing.

So, if you want to take the base of natural logarithms (e = 2.718....) and raise it to a complex power (e.g. a number that contains an imaginary part) you can figure out the answer by using sin and cosine, which any old calculator can do. Nice.

It leads to the astounding identity (truth) that is posted up there and that I want tattooed on my body. It simply and concisely links together 5 of the most fundamental and seemingly unconnected numbers on mathematics:

e = the base of natural logarithms, this describes things like radioactive decay and population growth.

i = the square root of minus one. The simplest complex number which can be seen as one of the fundamental tools in solving algebraic equations such as those that govern motion, gravity, pressure etc.

pi = the ratio of a circle's circumference to it's diameter.

1 = the basis of all counting

0 = the empty set, the absence of number, xen, innit?

Beautiful.

Without meaning to breeze over all this beautiful maffs...

Matt Black's work looks fucking awesome, good shout.

its Roy his bike, from Rotterdam, if you were in brussels the other day you could have seen it, otherwise it wasnot that bike

its a cutter right?
i saw him in 14 a couple of weeks ago.
i met liam sparkes the other day.
nice guy.

yeah its a cutter and we was in London a few weeks ago

its Roy his bike, from Rotterdam, if you were in brussels the other day you could have seen it, otherwise it wasnot that bike

Ha :)
Fair enough. Unless I saw it in a video and imagined I was there..
Or I dreamt it. Or it was some kind of vision of the future.

Or something.